Heat Flow through a Pipe
Heat Flow through a Pipe
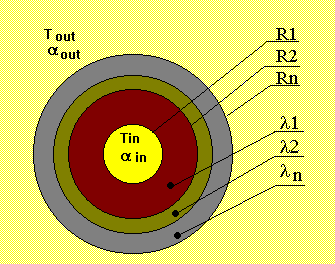
The heat transfer through a pipe is dependent on the thickness of the pipe
and isolation layers. The thickness of the pipe and layers can be defined by the radius of layers i.e. R1, R2,..., Rn.
The thermal conductivity of layers are,  1,
1,
 2,..., . The fluid within the pipe is at temperature Tin,
and the heat transfer coefficient from fluid to the wall is
2,..., . The fluid within the pipe is at temperature Tin,
and the heat transfer coefficient from fluid to the wall is
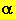 in.
The temperature and heat transfer coefficient for the fluid outside
the pipe are Tout and
in.
The temperature and heat transfer coefficient for the fluid outside
the pipe are Tout and 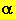 out. By using
Fourier's law of conduction and
Newton's law of cooling, it can be
shown that for a steady state heat transfer:
out. By using
Fourier's law of conduction and
Newton's law of cooling, it can be
shown that for a steady state heat transfer:
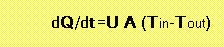
where,

dQ/dt= The transferred heat per unit time
L= Length of the pipe
A= 2*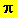 *L*R1
*L*R1
U which has the same unit as heat transfer coefficient is known as the overall
heat transfer coefficient.
Copyright © 1998 Taftan Data. All Rights Reserved
*L*R1